
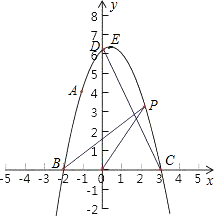
 ,与x轴分别交于B(x1,0)、C(x2,0)两点(其中且x1<x2),且x12+x22=13.
,与x轴分别交于B(x1,0)、C(x2,0)两点(其中且x1<x2),且x12+x22=13.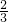 倍,求点P的坐标.
倍,求点P的坐标.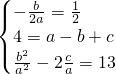 ?
?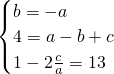 ?
?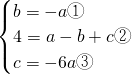
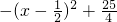
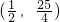
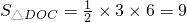
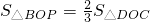 ,即
,即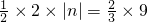
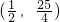 ;
; 列出关系式
列出关系式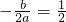 ;与x轴分别交于B(x1,0)、C(x2,0)两点(其中且x1<x2),且x12+x22=13,那么可得到方程ax2+bx+c=0,因此x1+x2=
;与x轴分别交于B(x1,0)、C(x2,0)两点(其中且x1<x2),且x12+x22=13,那么可得到方程ax2+bx+c=0,因此x1+x2= ,x1•x2=
,x1•x2=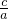 ,则利用完全平方式可得
,则利用完全平方式可得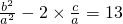 .联立三式组成方程组,可解得a、b、c的值,则抛物线的解析式即可确定.再将解析式写出顶点式,则顶点坐标E也就确定.
.联立三式组成方程组,可解得a、b、c的值,则抛物线的解析式即可确定.再将解析式写出顶点式,则顶点坐标E也就确定.

科目:初中数学 来源: 题型:
| c2 |
| 4 |
| 3 |
 存在过P、Q两点且与y轴相切的圆?若存在,求出圆的圆心坐标,若不存在,请说明理由.
存在过P、Q两点且与y轴相切的圆?若存在,求出圆的圆心坐标,若不存在,请说明理由.查看答案和解析>>
科目:初中数学 来源: 题型:
| c |
| a |
| 2 |
查看答案和解析>>
科目:初中数学 来源: 题型:
 (2013•贵阳)已知:直线y=ax+b过抛物线y=-x2-2x+3的顶点P,如图所示.
(2013•贵阳)已知:直线y=ax+b过抛物线y=-x2-2x+3的顶点P,如图所示.查看答案和解析>>
科目:初中数学 来源: 题型:解答题
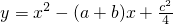 ,其中a、b、c是△ABC的∠A、∠B、∠C的对边.
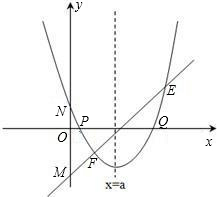
,其中a、b、c是△ABC的∠A、∠B、∠C的对边. ,抛物线与x轴交于点P、Q,问是否
,抛物线与x轴交于点P、Q,问是否 存在过P、Q两点且与y轴相切的圆?若存在,求出圆的圆心坐标,若不存在,请说明理由.
存在过P、Q两点且与y轴相切的圆?若存在,求出圆的圆心坐标,若不存在,请说明理由.查看答案和解析>>
科目:初中数学 来源:2009年四川省绵阳市南山中学自主招生考试数学试卷(解析版) 题型:解答题
 ,其中a、b、c是△ABC的∠A、∠B、∠C的对边.
,其中a、b、c是△ABC的∠A、∠B、∠C的对边.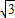 ,抛物线与x轴交于点P、Q,问是否存在过P、Q两点且与y轴相切的圆?若存在,求出圆的圆心坐标,若不存在,请说明理由.
,抛物线与x轴交于点P、Q,问是否存在过P、Q两点且与y轴相切的圆?若存在,求出圆的圆心坐标,若不存在,请说明理由.
查看答案和解析>>
湖北省互联网违法和不良信息举报平台 | 网上有害信息举报专区 | 电信诈骗举报专区 | 涉历史虚无主义有害信息举报专区 | 涉企侵权举报专区
违法和不良信息举报电话:027-86699610 举报邮箱:58377363@163.com