
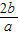 ,x1•x2=
,x1•x2=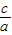 ,则可得d2=|x1-x2|2=(x1+x2)2-4x1•x2,又由a>b>c,a+b+c=0,得到函数f(
,则可得d2=|x1-x2|2=(x1+x2)2-4x1•x2,又由a>b>c,a+b+c=0,得到函数f(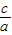 )=4[(
)=4[(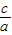 )2+
)2+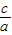 +1],根据其增减性即可求得答案.
+1],根据其增减性即可求得答案.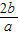 ,x1•x2=
,x1•x2= ,
,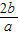 )2-
)2-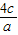 =
=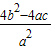 =
=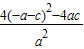 =4[(
=4[(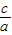 )2+
)2+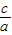 +1]=4[(
+1]=4[( +
+ )2+
)2+ ]
]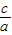 <-
<- ,
,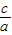 )=4[(
)=4[(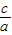 )2+
)2+ +1]的对称轴为:
+1]的对称轴为: =-
=- ,
,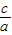 <-
<- 时,f(
时,f(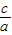 )=4[(
)=4[(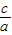 )2+
)2+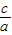 +1]是减函数,
+1]是减函数,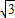 <d<2
<d<2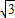 ,
,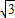 <|x1-x2|<2
<|x1-x2|<2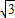 .
.

科目:初中数学 来源:不详 题型:填空题
查看答案和解析>>
湖北省互联网违法和不良信息举报平台 | 网上有害信息举报专区 | 电信诈骗举报专区 | 涉历史虚无主义有害信息举报专区 | 涉企侵权举报专区
违法和不良信息举报电话:027-86699610 举报邮箱:58377363@163.com