
科目:初中数学 来源: 题型:解答题
查看答案和解析>>
科目:初中数学 来源: 题型:选择题
| A. | 了解某班学生最喜爱的体育项目 | B. | 核实某位病人血液中被感染的病毒 | ||
| C. | 了解长江中鱼的种类 | D. | 调查一批炮弹的杀伤半径 |
查看答案和解析>>
科目:初中数学 来源: 题型:解答题
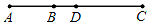 如图所示,点C在线段AB的延长线上,且BC=2AB,D是AC的中点.看图说话:
如图所示,点C在线段AB的延长线上,且BC=2AB,D是AC的中点.看图说话:查看答案和解析>>
科目:初中数学 来源: 题型:解答题
查看答案和解析>>
科目:初中数学 来源: 题型:解答题
查看答案和解析>>
湖北省互联网违法和不良信息举报平台 | 网上有害信息举报专区 | 电信诈骗举报专区 | 涉历史虚无主义有害信息举报专区 | 涉企侵权举报专区
违法和不良信息举报电话:027-86699610 举报邮箱:58377363@163.com