
科目:高中数学 来源:不详 题型:解答题
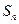 为数列
为数列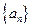 的前
的前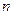 项和,对任意的
项和,对任意的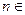 N
N ,都有
,都有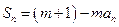
 为常数,且
为常数,且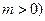 .
.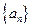 是等比数列;
是等比数列;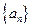 的公比
的公比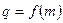 ,数列
,数列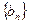 满足
满足
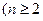 ,
,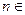 N
N
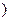 ,求数列
,求数列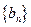 的通项公式;
的通项公式;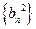 的前
的前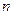 项和
项和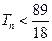 .
.查看答案和解析>>
科目:高中数学 来源:不详 题型:单选题
| A.2011 | B. |
| C.22011 | D.2-2011 |
查看答案和解析>>
科目:高中数学 来源:不详 题型:解答题
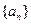 是一个等差数列,其前
是一个等差数列,其前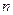 项和为
项和为 ,且
,且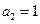 ,
,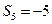 .
.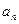 ;
; 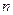 项和
项和 ,并求出
,并求出 的最大值.
的最大值.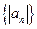 的前
的前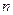 项和
项和 .
.查看答案和解析>>
湖北省互联网违法和不良信息举报平台 | 网上有害信息举报专区 | 电信诈骗举报专区 | 涉历史虚无主义有害信息举报专区 | 涉企侵权举报专区
违法和不良信息举报电话:027-86699610 举报邮箱:58377363@163.com