1、函数极限的概念

　(1)一般地，当自变量x取正值且无限增大时，如果函数y=f(x)的值无限趋近于一个常数A，就说当x趋向于正无穷大时，函数y=f(x)的极限是A，记作．

　(2)一般地，当自变量x取负值而|x|无限增大时，如果函数y=f(x)的值无限趋近于一个常数A，就说当x趋向于负无穷大时，函数y=f(x)的极限是A，记作．

　(3)一般地，当自变量x的绝对值无限增大时，如果函数y=f(x)的值都无限趋近于一个常数A，就说当x趋向于无穷大时，函数y=f(x)的极限是A，记作．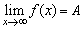

　(4)一般地，当自变量x无限趋近于常数x₀(但x≠x₀)时，如果函数y=f(x)无限趋近于一个常数A，就说当x趋向于x₀时，函数y=f(x)的极限是A，记作．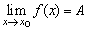

　说明：(1)我们常把(1)(2)称为函数的单侧极限，我们通常通过解析式或函数图象来判断函数单侧极限，函数的单侧极限不一定同时存在，例如，给定函数f(x)=2x，显然当x→+∞时，2^x→+∞，即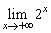不存在，但．

　(2)函数f(x)当x→x₀(或x→∞)时极限存在的必要且充分条件是：

　x→x₀⁺(或x→+∞)及x→x₀^－(或x→－∞)的极限各自存在并且相等，通常简述为：左、右极限存在并且相等．

　(3)当x→x₀时f(x)在x₀处是否有定义无关，因为x→x₀并不要求x=x₀．

　(4)若函数f(x)在x=x₀处及其附近有意义，且图象在x=x₀处不间断，则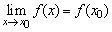．如．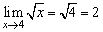

　本讲在上讲已经学习数列的极限的基础上来学习函数的极限，主要探讨函数极限的概念及函数极限的运算法则，学会求函数的极限．

8.(★★★★★)________,I have never seen anyone who’s as capable as John.

　　 A.As long as I have traveled

　　 B.Now that I have travelled so much

　　 C.Much as I have travelled

　　 D.As I have travelled so much

7.(★★★★★)So badly________ in the accident that he was sent to the nearest hospital immediately.

　　 A.he did injure B.he was injured

　　 C.did he injure D.was he injured

6.(★★★★)After that we never saw her again nor________ here.

　　 A.we heard B.have we heard

　　 C.we had heard D.did we hear

5.(★★★★★)-“It was cold yesterday.”

　　 -________.

　　 A.It was so B.So it was C.It did so D.So it did

4.(★★★★★)________that we can’t catch up with him.

　　 A.So fast did he run B.Fast as he ran

　　 C.Fast he ran so D.He ran fast

3.(★★★★★)Now________ to look after the wounded.

　　es my turn

　　 C.my turn came D.my turn is coming

2.(★★★★★)Autumn coming,down________.

　　 A.the leaves full B.leaves falling

　　 C.fall the leaves D.so the leaves full

1.(★★★★★)________,I would have told you the truth.

　　 A.If you asked me B.You had asked me

　　 C.Had you asked me D.Should you have asked me